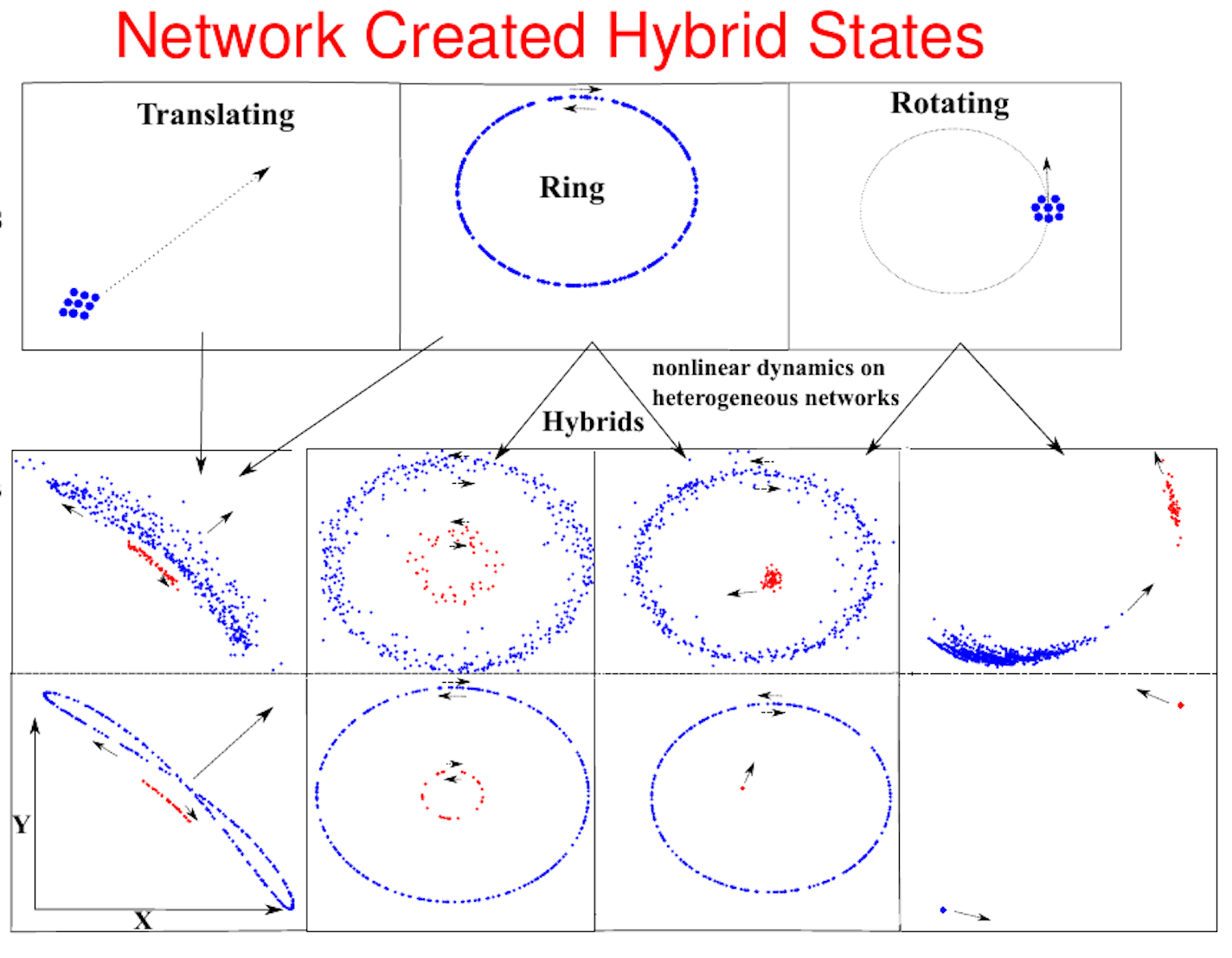
Emergent Pattern Forming Swarms and the Physics of Mixed-Reality

Swarming behavior continues to be a subject of immense interest because of its centrality in many naturally occurring systems in physics and biology, as well as its importance in engineering applications such as robotics. Here we examine the effects on coherent swarm pattern formation from aspects of communication, such as latency effects, link topology and environmental uncertainty.
With the availability of ever more cheap and powerful computing, interest in the use of mixed-reality and swarm experiments has grown considerably in the biological, engineering and physical sciences. Broadly speaking, these experiments consist of a simulated, or virtual model coupled directly to a physical experiment. Within the physical experiment, it is typical to find a good deal of uncertainty and noise since it is connected to the real world, and thus subjected to random perturbations. In contrast, the virtual part of the coupled system represents a somewhat idealized version of reality in which noise can be eliminated entirely. Thus, mixed-reality systems have very skewed sources of uncertainty spread through the entire system.
In this talk, we consider the pattern formation of delay - coupled swarms theoretically and experimentally to illustrate the idea of mixed-reality. Motivated by physical experiments, we then consider a model of a mixed-reality system, and show how noise in the physical part of the system can influence the virtual dynamics through a large fluctuation, even when there is no noise in the virtual components. We quantify the effects of uncertainty by showing how characteristic times of noise induced switching between swarm patterns scale as a function of the coupling between the real and virtual parts of the experiment. This work is done in collaboration with Klimka Szwaykowska, Thomas Carr, and Jason Hindes.